JAJSFB6D April   2018  – July 2022 INA180-Q1 , INA2180-Q1 , INA4180-Q1

PRODMIX  

  1. 1 特長
  2. 2 アプリケーション
  3. 3 概要
  4. 4 Revision History
  5. 5 Device Comparison
  6. 6 Pin Configuration and Functions
  7. 7 Specifications
    1. 7.1 Absolute Maximum Ratings
    2. 7.2 ESD Ratings
    3. 7.3 Recommended Operating Conditions
    4. 7.4 Thermal Information
    5. 7.5 Thermal Information
    6. 7.6 Electrical Characteristics
    7. 7.7 Typical Characteristics
  8. 8 Detailed Description
    1. 8.1 Overview
    2. 8.2 Functional Block Diagrams
    3. 8.3 Feature Description
      1. 8.3.1 High Bandwidth and Slew Rate
      2. 8.3.2 Wide Input Common-Mode Voltage Range
      3. 8.3.3 Precise Low-Side Current Sensing
      4. 8.3.4 Rail-to-Rail Output Swing
    4. 8.4 Device Functional Modes
      1. 8.4.1 Normal Mode
      2. 8.4.2 Input Differential Overload
      3. 8.4.3 Shutdown Mode
  9. 9 Application and Implementation
    1. 9.1 Application Information
      1. 9.1.1 Basic Connections
      2. 9.1.2 RSENSE and Device Gain Selection
      3. 9.1.3 Signal Filtering
    2. 9.2 Typical Application
      1. 9.2.1 Design Requirements
      2. 9.2.2 Detailed Design Procedure
      3. 9.2.3 Application Curve
    3. 9.3 Power Supply Recommendations
      1. 9.3.1 Common-Mode Transients Greater Than 26 V
    4. 9.4 Layout
      1. 9.4.1 Layout Guidelines
      2. 9.4.2 Layout Examples
  10. 10Device and Documentation Support
    1. 10.1 Documentation Support
      1. 10.1.1 Related Documentation
    2. 10.2 Receiving Notification of Documentation Updates
    3. 10.3 サポート・リソース
    4. 10.4 Trademarks
    5. 10.5 Electrostatic Discharge Caution
    6. 10.6 Glossary
      1.      Mechanical, Packaging, and Orderable Information

パッケージ・オプション

メカニカル・データ（パッケージ｜ピン）
サーマルパッド・メカニカル・データ
発注情報

9.1.2 RSENSE and Device Gain Selection

The accuracy of the INAx180-Q1 is maximized by choosing the current-sense resistor to be as large as possible. A large sense resistor maximizes the differential input signal for a given amount of current flow and reduces the error contribution of the offset voltage. However, there are practical limits as to how large the current-sense resistor can be in a given application. The INAx180-Q1 have a typical input bias currents of 80 µA for each input when operated at a 12-V common-mode voltage input. When large current-sense resistors are used, these bias currents cause increased offset error and reduced common-mode rejection. Therefore, using current-sense resistors larger than a few ohms is generally not recommended for applications that require current-monitoring accuracy. A second common restriction on the value of the current-sense resistor is the maximum allowable power dissipation that is budgeted for the resistor. Equation 1 gives the maximum value for the current sense resistor for a given power dissipation budget:

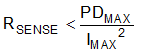
Equation 1. GUID-D22B6BBC-6DF1-468C-A29D-92424755A1B3-low.gif

where:

  • PDMAX is the maximum allowable power dissipation in RSENSE.
  • IMAX is the maximum current that will flow through RSENSE.

An additional limitation on the size of the current-sense resistor and device gain is due to the power-supply voltage, VS, and device swing to rail limitations. In order to make sure that the current-sense signal is properly passed to the output, both positive and negative output swing limitations must be examined. Equation 2 provides the maximum values of RSENSE and GAIN to keep the device from hitting the positive swing limitation.

Equation 2. GUID-65767108-C843-48B1-837C-E9B63FDC7536-low.gif

where:

  • IMAX is the maximum current that will flow through RSENSE.
  • GAIN is the gain of the current sense-amplifier.
  • VSP is the positive output swing as specified in the data sheet.

To avoid positive output swing limitations when selecting the value of RSENSE, there is always a trade-off between the value of the sense resistor and the gain of the device under consideration. If the sense resistor selected for the maximum power dissipation is too large, then it is possible to select a lower-gain device in order to avoid positive swing limitations.

The negative swing limitation places a limit on how small of a sense resistor can be used in a given application. Equation 3 provides the limit on the minimum size of the sense resistor.

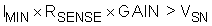
Equation 3. GUID-64D0427B-A6F9-4D60-B167-C7D9054021C9-low.gif

where:

  • IMIN is the minimum current that will flow through RSENSE.
  • GAIN is the gain of the current sense amplifier.
  • VSN is the negative output swing of the device (see Section 8.3.4).